How to wire speakers in series or parallel to an IP Former
In this knowledge base article we will explain the difference between series and parallel loudspeaker wiring and how the different configuration affect the power distribution
Understanding the specifications
Before talking about speaker wiring configuration, a bit of theory about amplifiers and speakers.
Audio amplifiers comes with a typical output power and impedance rating. I.e. 6W rms @ 4 Ω
This means that the amplifier is capable of delivering that amount of power with that particular load (impedance) connected to its terminals. Doubling the load will result in an inefficiency since it will halve the power that the amplifier is capable to deliver, viceversa, halving the impedance will require the amplifier to double the power.
The above statement is true for an “ideal” amplifier, which in theory is a perfect amplifier. In reality the measurements never show a double or a halve of the power output. But the concept is: reducing the impedance increases the power, while increasing the impedance reduces the power.
On the other side, speakers also come with an impedance and power handling rate (among many other characteristics not in the scope of this tutorial). The impedance of a speaker corresponds to its minimum “resistance” at a certain frequency, means that is the minimum the speaker can reach (thus the maximum current drain that will affect the amplifier), in fact the speaker impedance is different throughout the frequency spectrum, the nominal impedance is an average of the lowest impedance the speaker can reach. The speaker power handling is the maximum power a loudspeaker can handle. This number should always be equal or greater to what the amplifier is capable to deliver.
To get the best out of your amplifier is always recommended to match the impedance of the amp output and of the speaker connected.
Case 1:
Amplifier specs: 6W @ 4 Ω
Speaker specs: 8W / 4 Ω
In this case the match is perfect. The speaker will be able to handle the max power delivered by the amplifier.
Case 2:
Amplifier specs: 6W @ 4 Ω
Speaker specs: 8W / 8 Ω
In this case the speaker has a greater impedance, in such case the amount of power delivered by the amplifier will be lower. In a perfect amplifier, which doesn’t exist in the real world, the power will halve.
Case 3:
Amplifier specs: 6W @ 4 Ω
Speaker specs: 8W / 2 Ω
In this case the amplifier will be required to deliver more power because of the lower impedance of the speaker connected. Never do this. As per amplifier specifications, the max output power can already be reached at 4 ohms and is 6W. Halving the impedance will require the amp to deliver theoretically 12W, of which it is not capable of. In the best case the amplifier will shut itself down, the protection circuit kicks in (or the fuse will blow) and the amp will not brake, in the worst case, if there is no protection against such cases the amplifier will brake.
The conclusion: always make sure to connect the proper load to an amplifier output and make sure to check the power output, the impedance at which will deliver that output and on the speaker side the speaker impedance and the power handling to ensure the speaker will be capable of handling the power delivered by the amplifier. The best results are when there is an impedance match between the amplifier and the loudspeaker.
Series wiring
Now that we understand about impedance and output power let’s see what happens when we wire speakers in series. Juts like when we were studying about the ohm’s law back in school, wiring resistors in series will generate a total resistance that corresponds to the sum of each resistor value. Easy.
In the audio world, there is no difference, wiring speakers in series will result in a total impedance “seen” by the amplifier that corresponds to the sum of the impedance value of each speaker.
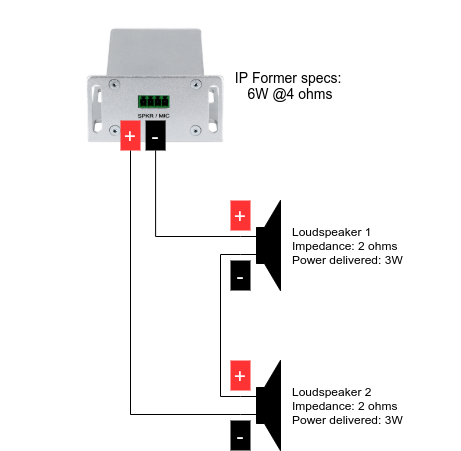
When wiring speakers with the same impedance in series the power distributed is equal to the power delivered by the amplifier (which will change according to the total impedance of the series circuit) divided by the number of speakers. An example in the diagram below:
In this scenario the total impedance is 4 Ω, which matches the IP Former optimal impedance for it to be able to deliver 6W of power. The power will be distributed evenly to both speakers, 3W each, thus the loudspeaker should be able to handle 3W of power or more.
If the impedance of each speaker is different the power will be distributed differently following, where the loudspeaker with the lower impedance will always get more power compared to the other in the circuit.
Parallel wiring
Wiring resistors in parallel produces a different result. If the each resistor wired in parallel has the same resistance value, the total resistance is equal to the individual resistance value / number of resistors in the circuit. Example:
R1 = 16 Ω
R2 = 16 Ω
R3 = 16 Ω
R4 = 16 Ω
Rtot = 16/4 = 4 Ω
So when wiring 4 speakers in parallel, having the same impedance, will result in a total impedance which is equal to the single impedance of each speaker divided by the number of speakers.
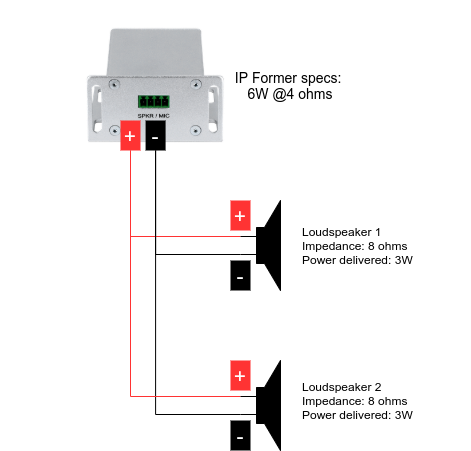
When wiring speakers with the same impedance in parallel the power distributed is equal to the power delivered by the amplifier (which will change according to the total impedance of the series circuit) divided by the number of speakers. An example in the diagram below:
In the above scenario the total impedance is 4 Ω since the 2 speakers are 8 Ω each. The power distributed is the same across both speakers because they have the same impedance.
If in a parallel circuit the impedance of each speaker is different the calculation to get the total impedance is different. In this case the total impedance is equal to: 1/((1/Ωspkr_1)+(1/Ωspkr_2)+(1/Ωspkr_n))
In this case the power distributed will change according to the impedance of each individual speaker.
At this link there is a useful calculator that can be used to verify how an ideal amplifier distributes the power across speakers wired in parallel plus some other useful resources that helps you in learning more about audio distributions in general.